Bar modelling is often credited as a central pillar in the successful mathematics curriculum and pedagogy of Singapore and other East Asian nations. Furthermore, the Mastery approach evolving in the UK has led to an increased focus on bar modelling and the CPA method.The TES has several excellent articles on this. More recently, New Zealand schools have shown a growing interest in this method as a tool for developing learner agency and accessing problem solving. But, when and how should be introduced?
Is there a place for bar modelling in early maths?
As children move towards the abstraction of using numerals, using the bar model can help children combine the ordinal and cardinal aspects of number. In the example below, the brace/bracket used in bar modelling labels the number of objects. This could be a technique adults might use and model to children during early counting experiences.
Teachers familiar with Martin Hughes' seminal work might suggest the example above is too big a conceptual leap for children without one-to-one correspondence. His work suggested that children used pictographic and iconic marks before the symbolic use of numerals. The examples below show how children might label amounts of objects pictographically or iconically before moving to using numerals.
 |
| An example of iconic labelling |
 |
| An example of pictographic labelling |
 |
| Developing 1-1 correspondence |
It could be argued that these images and experiences are not different to any early years classroom. Giving children rich experiences of counting, classification, sorting and identifying pattern are cornerstones of early mathematical experience. I'd argue that the bracket or brace encompassing the objects that are being counted is very powerful. I believe that this helps to cement the cardinal aspect of number, consolidating the 'threeness' of the objects.
Look at the image below, if we were to ask the question, 'where is the 3?' Would children point to the 3rd train on the right (assuming they have counted from left to right)? The brace helps us to show that once we have counted the third cube (whichever cube that may be) we know the number in the set.
The next step for young students is to begin bar modelling. Again, we encourage children to build on their counting experiences and manipulate concrete apparatus. Here the model is discrete, and still encourages and supports one-to-one matching. This also highlights the abstraction principle of counting. Children begin to see that they can count the pictorial representation of the block rather the block itself.
 |
| A discrete bar model |
Developing counting strategies, calculation and understanding of pattern
The next step in the progression is to move from a model of 3 ones to a continuous model of one group of 3. Conceptually this is a significant change in understanding. I'd argue that it isn't sufficient to do this only by bar modelling. Educators need to consider whether they have grouped objects for students to explore. This is where Numicon and Cuisenaire are so powerful and, for me, crucial maths resources for teaching.
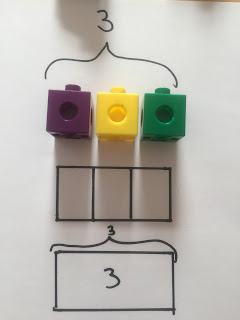 |
| Moving from a discrete model to a continuous model |
Now that the 3 the in photograph above has been unitized we can work with it. So, we could place another 3 alongside it and begin thinking about multiplication as repeated addition. First though, we are developing counting strategies. In the calculation, '3+2' a developmental step would be to count all of the objects (or blocks in the bar) but now the 3 is continuous children have a scaffold to help them count on from 3, rather than begin by counting the objects within the 3.
 |
| The bar model to count on |
The next step would be to draw two continuous bars labelled 3 and 2 next to each other. At this point, I'd ask educators to consider how they move children away from the developmental stage of counting to calculate. The question could be rephrased as, 'Which manipulatives are you using to secure knowledge of number facts?' Numicon, Cuisenaire, tens frames and bead strings, all offer children a variety of experiences with number. Personally, I feel that Numicon is the most powerful - I'll save that blog for another day.
Where next with Bar modelling?
Once children have experience developing their additive reasoning with concrete apparatus and bar models, they can then be used to develop (or assess) their multiplicative understanding. Starting to use bar modelling and its principles early on, will mean students are familiar with it and its conventions for different aspects of number.
Bar modelling for young children - how is it beneficial?
Hopefully, this has answered some of your questions regarding using the bar. My previous post discusses bar modelling as a problem solving tool for increasing learner agency. Nick Hart's blog is also an excellent resource.
How do I get this into my classroom?
At first, including bar modelling in your classroom can be quite daunting. As with any new pedagogy, it is important to understand the principles behind the method. Sign up to a training course!
UK educators, go here. The White Rose training is exceptional. NZ teachers, you can access the same training by attending a CPA Maths workshop







No comments:
Post a Comment